
| Braid Theory |
 10102 as a closed braid |
Braid theory was invented in 1925 by Emil Artin and
has developed semi-independently of knot theory.
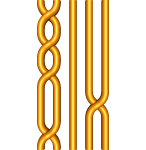
We can think of a braid as a special type of knot diagram in
which there are N equally spaced points connected via
N strands to a second set of N points as in the examples shown below
for N equal 2 to 6:
 |
 |
 |
 |
 |
A further requirement is that the strands proceed monotonically downward. If we draw a series of horizontal lines in the plane between the two sets of points, it is not difficult to see that the braid can always be drawn such that between any pair of horizontal lines there is only one crossing of the form
 , ,
s1 |
 , ,
s2 |

|

sn - 1 |
 , ,
s1-1 |
 , ,
s2-1 |

|

sn - 1-1 |
It is clear that any braid may be constructed by using these generators and the operation of composition.